Card Counting the Big Tiger Baccarat Side Bet
An AP recently contacted me and asked if I would analyze the “Big Tiger” baccarat side bet. This side bet pays 50-to-1 if the Banker wins with a three-card total of six, otherwise it loses. This is very similar to the infamous Dragon 7 (aka Fortune 7), which pays 40-to-1 if the Banker wins with a three-card total of seven. But there’s a big difference right away. The Dragon 7 has an off-the-top house edge of 7.611%, but the Big Tiger has a house edge of 15.253%. That’s enough right there to make the Big Tiger virtually worthless to the AP.
Here is the combinatorial analysis:
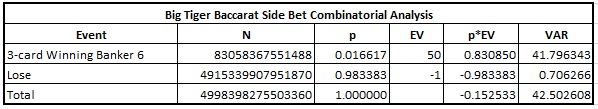
In particular:
- The house edge is 15.2533%.
- The standard deviation (square root of variance) is 6.519.
- The hit percentage is 1.662%.
I did the usual thing, compute the EOR’s and develop a card counting system. The system I came up with is not that great, it only has a betting correlation of 0.981 (I strive for > 0.99). But, it’s easy enough and there is no balanced system that is obviously better.
Here it is:
- A = 0
- 2 = 0
- 3 = -1
- 4 = -1
- 5 = -1
- 6 = -2
- 7 = +1
- 8 = +2
- 9 = +2
- T, J, Q, K = 0
You can intuitively see that this makes sense. First, if the Banker is going to draw a third card, it helps if both a natural 8 and 9 are less likely, hence the +2 tag for 8’s and 9’s. Drawing a 7 can never make a three-card total of 6, hence the +1 on that. As for the tags on the 3, 4, 5, 6, those are great draw cards for the Banker to make his three-card six, hence the negative values when those leave the shoe.
I ran a simulation of one hundred million (100,000,000) eight deck shoes, with the cut card at 14 cards, using the count system above. Here are the results:
- Target true count: +8
- Bet frequency: 3.536%
- Average edge: 7.227%
- Units won per 100 hands: 0.256
- Desirability index: 2.08
That’s all pretty miserable stuff as far as beating this bet. My opinion is that this wager does not pose a significant opportunity for the AP. I would rank the vulnerability of this side bet well into the “minimal” range.
